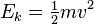T-80 Tanks

Well, I think you can just use force divided by the cross-section of the barrel to calculate the pressure. (This is by assuming that the pressure is the same in all direction everywhere in the barrel.)

I think a better way to calculate the pressure is to use the adiabatic expansion (no heat exchange between the gas in the barrel and the surrounding) of the ideal gas. Then we know that Pressure*(Volumn^gamma)=constant, which gives us a relation between pressure and volume. Then integrating Pressure*dVolume from the start to the end of the barrel will give us the kinetic energy obtained by the round. This will help fixing the initial pressure in the barrel. (Roughly speaking, as the volume of the gas increases, the pressure decreases in the barrel as the round moves forward, the temperature of the gas decreases, and the gas looses internal energy, which is converted to the kinetic energy obtained by the round.)

This is as far as I can go. If someone here knows the correct initial condition (pressure, volume or temperature) then he/she can continue further…

Click to expand...

Yeah, I know I did a very crude est about the pressue, If there is any really good programmer here, he/she can write a simple program to simulate the whole process with better data, a seasoned programmer can do this is about an hour and have a much accurate result. What I did was just pointing out the fact, moving small object faster and over a longer distance will add that much strain to the barrel than moving a heavier object slower and over a shorter distance, as long as their KE is about the same and no NRG was lost(imposible, i know). And the number are just there to make it more clear.

fishhead said:

1.
You estimate never comes with any mathematic prove, so I can't take your words as its face value. It has no credit for me.

2.
The penetrating power not only depends on the mass, also the L/D ratio impacts a lot on it, as well as speed. Although I don't know exactly the relationship, I don't think using only mass is right.

3.
All M829 family have very low speed, so don't use them to compare with Chinese shell.

Don't believe too much of your propaganda, here is the official DOD document: All M829 muzzle speeds are 15xx m/s something, way lower than Chinese 1780m/s.

Please, Log in or Register to view URLs content!

You M829E3 is still in test stage, so don't use it to compare. Even that we know it has a low speed, 15xx m/s something.

Please, Log in or Register to view URLs content!

Stop using Russian or American shells to compare with Chinese round.

Click to expand...

I just spoke with someone with General Dynamics Ordinance and Tactical Systems Canada (formerly known as SNC-TEC) about this issue, and this is what he had to say to me:

1. There is no way from a physics standpoint that the Chinese 125mm gun system will have more kinetic energy than the NATO 120mm gun system with a lighter round, due to a higher muzzle velocity from current information. There would have to be a significant improvement in metallurgy in both the gun itself, and with the round to achieve those higher muzzle velocities due to the higher pressures exerted to achieve those higher velocities. Likewise, there will have to be a significant advancement of propellant technology beyond what is currently known.

2. To calculate KE (penetrating power) is basic high school physics, as it is this formula used:

where m is the mass (in kg) and v is the velocity (in meters per second) of the body. For the Chinese round to achieve a higher amount of kinetic energy with a lighter round (mass), you will need a substantial increase in kinetic energy. L/D ratio is NOT INVOLVED HERE.

3. The M829 family of munitions has a much higher mass, which means despite the slightly lower muzzle velocity, the M829 family of munitions still has a high amount of Kinetic energy due to the increased mass of the round.

4. It is a very valid comparison, comparing Russian and Western rounds to Chinese rounds. Western rounds have been proven in combat, and their performance has been demonstrated.

Fishhead,

the m829e3 is in service and my math does check becuase it is mostly based off your figures for speed and L/D ratio.

the DM 53 uses 31/1 ratio the chinese round uses 30/1 so it is safe to assume the 829 also uses a 30/1 or there abouts L/D ratio based on mass.

The 829 and DM53 both come in between 11,000j per mm of RHAe and 11,900 per mm of RHAe so I split the differance between the 2 and assigned the Chinese round a penetration power of 11,445j per mm of RHAe

Then I did real basic mass 11,445*960= 10,987,200j since you provided velocity it was simple trial and error to find a projectile weight that came in at the proper figure. That figure happened to be 9kg.

based on the listed weight of the spool sabot on the 3BM42 i subtracted 2.2kg from each of the other three rounds. All 4 rounds have similar bore sizes and all use a spool type sabot and becuase the weight was applied uniformly across the board it won't skew the results vs any other round.

Granted the weight of the spool might be off by a small amount of the efficency of the round might be better by a small amount but the numbers fit and they fit by comparison. You might not like it but you don't have too, the numbers fit.

At this point I am willing to concede the PLA may have sacraficed economy inorder to achieve a high leve of KE. Wether or not the round can actually retain its shape in combat vs modern armors is another story, hopefully we never find out.

Now will the Chinese gun work vs a M1A2SEP level of protection? maybe, tank net estimates give the Abrams M1A2SEP a maximum frontal armor of 960mm RHAe vs KE so the Chinese round has the power to penetrate if it hits a weaker area but will probalby lose most of its energy.

The 829E3 however has energy left over no matter where it hits the ztz-99. Which is estimated to have a protection level of 800mm RHAe. Plus the ztz-99 has uber deadly hull stoage carousel autoloader.



The reason I sue the A2 levels of protection and the 829 is the new SK2. It should have protection levels equal to anything in the world was designed in the same theater as China and has a 120mm L/55. It is now the dominant tank in Asia (besides the US Army's small tank force in Korea and Japan) and so now must be considered as the ZTZ-99's primary rival in the area (besides the T-90, T-90S, and T-80UD)

Pointblank said:

I just spoke with someone with General Dynamics Ordinance and Tactical Systems Canada (formerly known as SNC-TEC) about this issue, and this is what he had to say to me:

1. There is no way from a physics standpoint that the Chinese 125mm gun system will have more kinetic energy than the NATO 120mm gun system with a lighter round, due to a higher muzzle velocity from current information. There would have to be a significant improvement in metallurgy in both the gun itself, and with the round to achieve those higher muzzle velocities due to the higher pressures exerted to achieve those higher velocities. Likewise, there will have to be a significant advancement of propellant technology beyond what is currently known.

Click to expand...

The KE is not only related to the mass, also the speed. Actually US shell speed is quite low. And we don't know the Chinese shell's mass, no reliable data.

Pointblank said:

2. To calculate KE (penetrating power) is basic high school physics, as it is this formula used:
[qimg]http://upload.wikimedia.org/math/2/3/1/231cfd9416f4736f5ee8d102ee84cb22.png[/qimg]
where m is the mass (in kg) and v is the velocity (in meters per second) of the body. For the Chinese round to achieve a higher amount of kinetic energy with a lighter round (mass), you will need a substantial increase in kinetic energy. L/D ratio is NOT INVOLVED HERE.

Click to expand...

So speed does contribute more, since it has a ^2 on it. L/D ration has nothing to do with KE, but a lot to do with the penetrating power as I said. Many research have been done it already.

Pointblank said:

3. The M829 family of munitions has a much higher mass, which means despite the slightly lower muzzle velocity, the M829 family of munitions still has a high amount of Kinetic energy due to the increased mass of the round.

Click to expand...

Its low speed reduces its KE a lot. 10% of speed drop leads to 21% reduce of KE.

Pointblank said:

4. It is a very valid comparison, comparing Russian and Western rounds to Chinese rounds. Western rounds have been proven in combat, and their performance has been demonstrated.

Click to expand...

You need data to prove it, not just statements.

zraver said:

Fishhead,

the m829e3 is in service and my math does check becuase it is mostly based off your figures for speed and L/D ratio.

the DM 53 uses 31/1 ratio the chinese round uses 30/1 so it is safe to assume the 829 also uses a 30/1 or there abouts L/D ratio based on mass.

The 829 and DM53 both come in between 11,000j per mm of RHAe and 11,900 per mm of RHAe so I split the differance between the 2 and assigned the Chinese round a penetration power of 11,445j per mm of RHAe

Then I did real basic mass 11,445*960= 10,987,200j since you provided velocity it was simple trial and error to find a projectile weight that came in at the proper figure. That figure happened to be 9kg.

Click to expand...

Your assumption has some flaw here, let me quote the original text:

"when firing third generation tungsten alloy APFSDS(initial speed 1,780m/sec), it could penetrate 850mm RHA at a distance of 2,000m. the latest special alloy armor-pieceing round can penetrate up to 960mm, the rod penetrator's length-diameter ratio is 30:1."

So the L/D ratio of 30:1 only applies to the "special alloy", aka DU here. It doesn't say what muzzle speed of DU shells, but gives the RHA of 960mm.

For tungsten shell, it gives the muzzle speed and penetrating depth of 850mm, but doesn't specify the L/D ratio.

You mix the two up, and we don't know which shell is in the photo.

@zraver: Reading your previous posts I suspect that your argument is partially derived from the flawed assumption that China's industrial tech level is quite primitive.
This was indeed the case may be 10 or 15 years ago but today e.g. China's steel industry has already replaced Japan's as the worlds leading producer of stainless, heat resistant and special steel in '06.Regarding the level of metallurgical science in China it is safe to assume that China has currently reached the russian level (one of the few fields the russian's are rather good!) and approaches korean and japanese tech leaders.

The new canon for ZTZ-99 is produced with the most modern technology China is able to apply and the gun is way better than the stuff the russian's are currently churning out for export (but indeed they have something up the sleeves exclusively for their own tank guards ). Of course China is not even near the magical capabilities :roll: of ´western´120 mm guns but since the Israelis have got their hands on the Rheinmetall gun a long time ago we should not be very much surprised if China has already managed that feat too. (... $$$ are not in short supply :roll: )

Just my five cents again:

1. Max barrell pressure is achieved early in the firing, not when the projectile reaches the muzzle. I understand why you use the barrell length when calculating pressure, but this doesn`t give us any real info. It does ofcourse depend on propellant combustion, but pressure should peak early and then get lower as the projectile moves down the barrell.
I think you are, though showing some fine math, going down a blind alley.

2. Fishhead: a light, high velocity projectile loses its energy far faster than a heavier one, given the same Ke0. This gives bad long range performance. Not fun

and quote: Its low speed reduces its KE a lot. 10% of speed drop leads to 21% reduce of KE.
This is wrong. If you try to calculate from to different speeds, you will see that

Violet Oboe said:

@zraver: Reading your previous posts I suspect that your argument is partially derived from the flawed assumption that China's industrial tech level is quite primitive.
This was indeed the case may be 10 or 15 years ago but today e.g. China's steel industry has already replaced Japan's as the worlds leading producer of stainless, heat resistant and special steel in '06.Regarding the level of metallurgical science in China it is safe to assume that China has currently reached the russian level (one of the few fields the russian's are rather good!) and approaches korean and japanese tech leaders.

The new canon for ZTZ-99 is produced with the most modern technology China is able to apply and the gun is way better than the stuff the russian's are currently churning out for export (but indeed they have something up the sleeves exclusively for their own tank guards ). Of course China is not even near the magical capabilities :roll: of ´western´120 mm guns but since the Israelis have got their hands on the Rheinmetall gun a long time ago we should not be very much surprised if China has already managed that feat too. (... $$$ are not in short supply :roll: )

Click to expand...

Why is it safe to assume that you have reached that level? Mocking other people views, and then coming with your own unsubstantiated biased views seems a bit silly.
And again, when we are discussing the claims made about the gun, just stating that it is way better than the russian guns seems like a party line. (communist party that is)

Pointblank said:

I just spoke with someone with General Dynamics Ordinance and Tactical Systems Canada (formerly known as SNC-TEC) about this issue, and this is what he had to say to me:

1. There is no way from a physics standpoint that the Chinese 125mm gun system will have more kinetic energy than the NATO 120mm gun system with a lighter round, due to a higher muzzle velocity from current information. There would have to be a significant improvement in metallurgy in both the gun itself, and with the round to achieve those higher muzzle velocities due to the higher pressures exerted to achieve those higher velocities. Likewise, there will have to be a significant advancement of propellant technology beyond what is currently known.

Click to expand...

this is my feeling as well. While there are indeed 2 variables in the KE equation, one of them is more or less fixed.

@skorzeny: In my post I made a certain distinction between russian export stuff and their MBT guns for ´home use´. China has acquired new technologies through various channels and regarding metallurgical R&D chinese universities and institutes have published more scientific articles and registered more patents in the last years than their russian counterparts. Of course that does not mean that China has currently nothing to learn from russian experts but actually a lot of special know how has been absorbed in the last 5-7 years. Unfourtunately some people harbour prejudices about ´primitive chinese copycats´ but the harsh reality of tough chinese competition has convinced them inevitably otherwise year after year.:coffee: